How To Calculate The Number Of Moles Of Gas Present

The ideal gas law can be used to derive a number of convenient equations relating directly measured quantities to properties of interest for gaseous substances and mixtures. Appropriate rearrangement of the ideal gas equation may be made to permit the calculation of gas densities and molar masses. Dalton's law of partial pressures may be used to relate measured gas pressures for gaseous mixtures to their compositions. Avogadro's law may be used in stoichiometric computations for chemical reactions involving gaseous reactants or products. For Equation 10.27 to be valid, the identity of the particles present cannot have an effect. Thus an ideal gas must be one whose properties are not affected by either the size of the particles or their intermolecular interactions because both will vary from one gas to another.

The calculation of total and partial pressures for mixtures of gases is illustrated in Example 11. The thin skin of our atmosphere keeps the earth from being an ice planet and makes it habitable. In fact, this is due to less than 0.5% of the air molecules. Of the energy from the sun that reaches the earth, almost \frac[/latex] is reflected back into space, with the rest absorbed by the atmosphere and the surface of the earth. Some of the energy that the earth absorbs is re-emitted as infrared radiation, a portion of which passes back out through the atmosphere into space. However, most of this IR radiation is absorbed by certain substances in the atmosphere, known as greenhouse gases, which re-emit this energy in all directions, trapping some of the heat.

This maintains favorable living conditions—without atmosphere, the average global average temperature of 14 °C (57 °F) would be about –19 °C (–2 °F). The major greenhouse gases are water vapor, carbon dioxide, methane, and ozone. Since the Industrial Revolution, human activity has been increasing the concentrations of GHGs, which have changed the energy balance and are significantly altering the earth's climate . As an example, equal volumes of gaseous hydrogen and nitrogen contain the same number of atoms when they are at the same temperature and pressure, and observe ideal gas behavior. In practice, real gases show small deviations from the ideal behavior and the law holds only approximately, but is still a useful approximation for scientists. If we know the volume, pressure, and temperature of a gas, we can use the ideal gas equation to calculate how many moles of the gas are present.

If we know how many moles of a gas are involved, we can calculate the volume of a gas at any temperature and pressure. As a reminder, gram molecular volume refers to the volume of one mole of a gas at a standard temperature and pressure . Temperature and pressure also play a major role. 1 atm and 273K are the standard values for pressure and temperature.

The ideal gas equation is used to get the gram molar volume, 22.4L. The pressure exerted by each gas in a gas mixture is independent of the pressure exerted by all other gases present. Consequently, the total pressure exerted by a mixture of gases is the sum of the partial pressures of the components (Dalton's law of partial pressures). The amount of gas present in a mixture may be described by its partial pressure or its mole fraction. The mole fraction of any component of a mixture is the ratio of the number of moles of that substance to the total number of moles of all substances present. In a mixture of gases, the partial pressure of each gas is the product of the total pressure and the mole fraction of that gas.

Thus, we can rearrange terms in the ideal gas equation and write them like this . Nevertheless, related experiments with some inorganic substances showed seeming exceptions to the law. This apparent contradiction was finally resolved by Stanislao Cannizzaro, as announced at Karlsruhe Congress in 1860, four years after Avogadro's death. He explained that these exceptions were due to molecular dissociations at certain temperatures, and that Avogadro's law determined not only molecular masses, but atomic masses as well. The molar mass of a particular gas is therefore equal to the mass of a single particle of that gas multiplied by Avogadro's number (6.02 x 1023 ). To find the molar mass of a mixture of gases, you need to take into account the molar mass of each gas in the mixture, as well as their relative proportion.

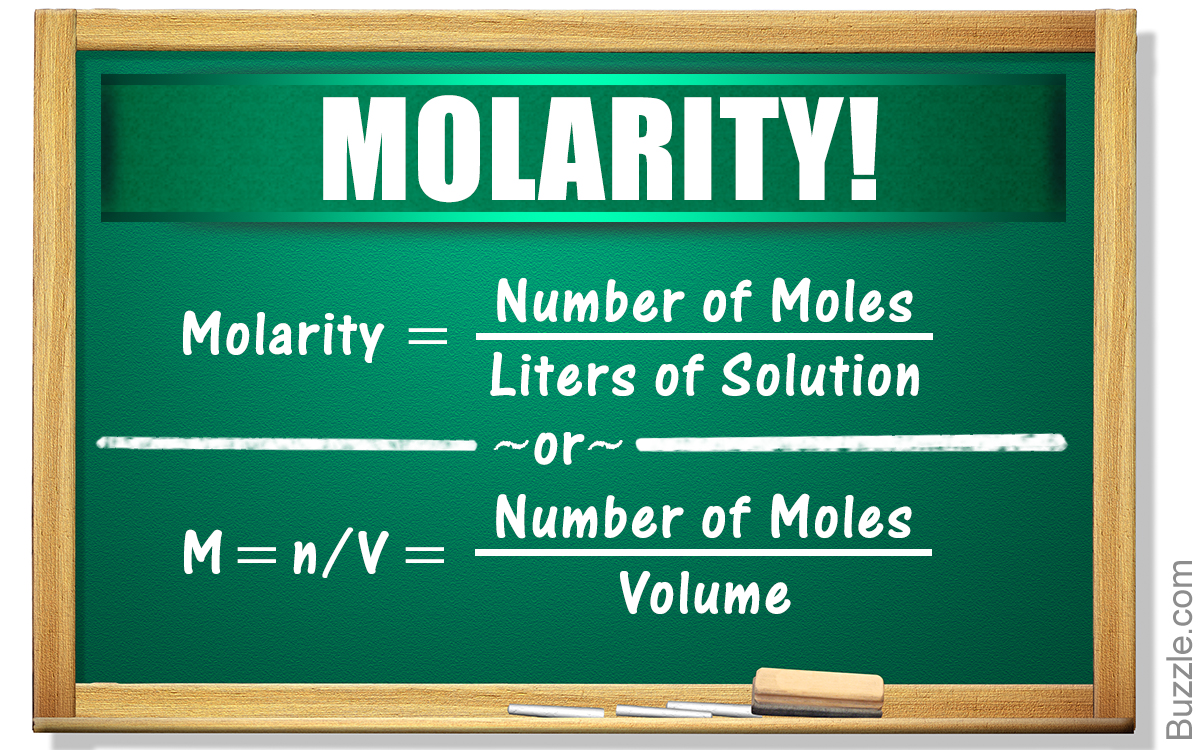
This chapter examines the concentration and kinetics in physiology. The concentration of a solute in solution is the amount of that solute per unit volume of solution. It can be expressed as the mass of the solute per unit volume or the number of moles of solute per unit volume.

A mole of any substance is Avogadro's number of particles. The relationship among concentration, amount of solute, and volume of solution can be used to determine the volume of physiological fluids. Evans' Blue Dye is an example of a solute that can be used to estimate plasma volume, because the dye enters the plasma but cannot leave it easily. Elementary chemical reactions have forward and reverse rate constants that govern the rate of conversion in either the forward or reverse reaction. The rates of reaction have the units of moles per unit time per unit volume of solution. Enzymes speed chemical reactions by altering the path of the reaction by allowing it to proceed on the surface of the enzyme.

Thus, enzymes convert homogeneous reactions in the fluid phase into heterogeneous reactions on the surface of the enzyme. It is found that the alternate path reduces the activation energy for the reaction, thereby allowing it to proceed quicker. Previously, we considered only ideal gases, those that fit the assumptions of the ideal gas law.

Gases, however, are never perfectly in the ideal state. All atoms of every gas have mass and volume. When pressure is low and temperature is low, gases behave similarly to gases in the ideal state. When pressure and temperature increase, gases deviate farther from the ideal state. We have to assume new standards, and consider new variables to account for these changes.

A common equation used to better represent a gas that is not near ideal conditions is the van der Waals equation, seen below. To get to moles, use the equation and the molar ratios shown. To get to volume, use the molar volume of gas constants. To get to mass, use the atomic/molecular masses shown in the periodic table. A mole of a pure substance is a mass of the material in grams that is numerically equal to the molecular mass in atomic mass units .

One mole of an ideal gas will occupy a volume of 22.4 liters at STP (Standard Temperature and Pressure, 0°C and one atmosphere pressure). When a reaction produces a gas that is collected above water, the trapped gas is a mixture of the gas produced by the reaction and water vapor. Water evaporates and there is always gaseous water above a sample of liquid water. At room temperatures, collisions between atoms and molecules can be ignored. In this case, the gas is called an ideal gas, in which case the relationship between the pressure, volume, and temperature is given by the equation of state called the ideal gas law.

To measure the volume of one mole of a gas at standard temperature and pressure , we use the gram molar volume . Since the gram molecular mass of an ideal gas is fixed at STP, temperature and pressure are the primary determinants of gram molecular mass. The standard temperature is 273 degrees Kelvin, and the standard pressure is one atmosphere. Hydrogen Hydrogen is the lightest gas in the mixture.

If equal masses of each gas are placed in the container, that would mean that hydrogen gas is present in the greatest number of moles . Therefore, when calculating partial pressures, the hydrogen gas would have the largest mole fraction and therefore the largest partial pressure. Partial pressure is a _________ because pressure results from the collisions of all the gas molecules with the walls of the gas container. The number density of the gas in container A is twice the number density of the gas in container B.

From the ideal gas law we know that pressure is governed by the number density of a gas sample. Container A has twice the pressure which means it must have twice the number density of gas as container B. Furthermore, since the volumes are identical we could say that container A has twice the gas moles as container B.

6When applied to gases, the molar volume of any gas is defined as occupying 22.4 dm3 at a temperature of 273 K and pressure 101.3 kPa . Volumes of gases are easier to measure than masses. Using the molar volume definition, if the volume of a gas is known, the number of moles and hence the mass of the gas can be determined. For example, to find the number of moles of carbon dioxide gas which are contained in 100 cm3 of the gas measured at 273 K and 101.3 kPa. Use is made of the above definition that at 101.3 kPa and 273 K, cm3 of any gas is the volume of 1 mole of the gas.

As we stated earlier, the shape of a gas is determined entirely by the container in which the gas is held. Sometimes, however, the container may have small holes, or leaks. Molecules will flow out of these leaks, in a process called effusion. Because massive molecules travel slower than lighter molecules, the rate of effusion is specific to each particular gas. We use Graham's law to represent the relationship between rates of effusion for two different molecules.

This relationship is equal to the square-root of the inverse of the molecular masses of the two substances. The molar volume of gas at STP, standard temperature and pressure (0°C or 273K, 100 kPa pressure) is 22.4 litres per mole (22.4 L/mol). In other words, one mole of atoms of a pure ideal gas at 0°C will fill 22.4 litres of space. The molar volume of gas at room temperature (25°C, 298K) and pressure is 24 litres per mole (24 L/mol). The ideal gas law is an equation of state the describes the behavior of an ideal gas and also a real gas under conditions of ordinary temperature and low pressure. This is one of the most useful gas laws to know because it can be used to find pressure, volume, number of moles, or temperature of a gas.

B Use the ideal gas law to calculate the partial pressure of each gas. Then add together the partial pressures to obtain the total pressure of the gaseous mixture. The explanation for this is illustrated in Figure 5. According to Avogadro's law, equal volumes of gaseous N2, H2, and NH3, at the same temperature and pressure, contain the same number of molecules. Calculate the number of moles of hydrogen gas present in \[500c\] of the gas taken at 300 \[K\] and 760 $mm$ pressure. If these samples of hydrogen were found to have a mass equal to \[4.09 \times g\] calculate the molar mass of hydrogen.

The ideal gas law can be considered to be another manifestation of the law of conservation of energy . Work done on a gas results in an increase in its energy, increasing pressure and/or temperature, or decreasing volume. This increased energy can also be viewed as increased internal kinetic energy, given the gas's atoms and molecules. Use the ideal gas law to calculate pressure change, temperature change, volume change, or the number of molecules or moles in a given volume. This is the ratio of the specific heat at constant pressure to that at constant volume.

Again it is a function of gas composition and static temperature, but total temperature may be used when the Mach number is less than 0.4. Gamma appears extensively in the "perfect gas" formulae relating pressure and temperature changes and component efficiencies. This means equal amounts of moles of gases occupy the same volume under the same conditions of temperature and pressure. Avogadro's law provides a way to calculate the quantity of gas in a receptacle.

Thanks to this discovery, Johann Josef Loschmidt, in 1865, was able for the first time to estimate the size of a molecule. His calculation gave rise to the concept of the Loschmidt constant, a ratio between macroscopic and atomic quantities. In 1910, Millikan's oil drop experiment determined the charge of the electron; using it with the Faraday constant , one is able to determine the number of particles in a mole of substance. At the same time, precision experiments by Jean Baptiste Perrin led to the definition of Avogadro's number as the number of molecules in one gram-molecule of oxygen. Perrin named the number to honor Avogadro for his discovery of the namesake law. Later standardization of the International System of Units led to the modern definition of the Avogadro constant.

We have just calculated the partial pressures of the major gases in the air we inhale. Experiments that measure the composition of the air we exhale yield different results, however. The following table gives the measured pressures of the major gases in both inhaled and exhaled air. Calculate the mole fractions of the gases in exhaled air. In our use of the ideal gas law thus far, we have focused entirely on the properties of pure gases with only a single chemical species. But what happens when two or more gases are mixed?

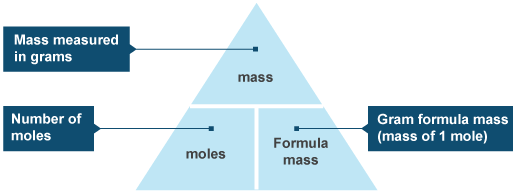
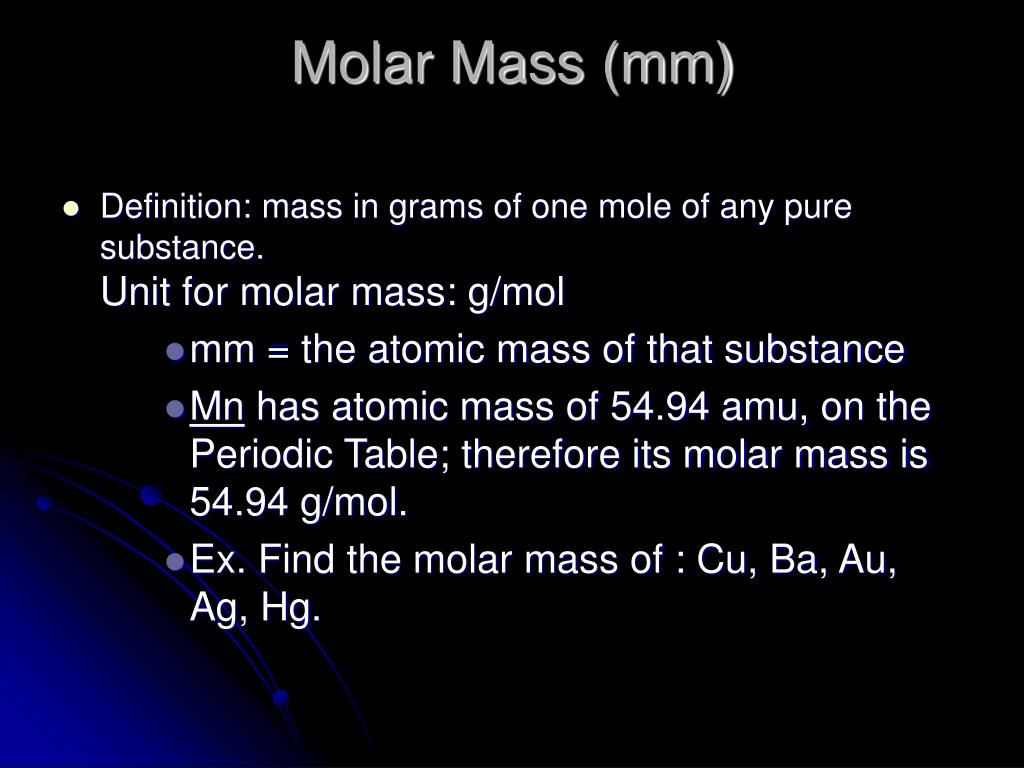
In this section, we describe how to determine the contribution of each gas present to the total pressure of the mixture. The ideal gas law relates the pressure and volume of a gas to the number of gas molecules and the temperature of the gas. One mole always contains 6.02 × 1023 particles , independent of the element or substance. A mole of any substance has a mass in grams equal to its molecular mass, which can be calculated from the atomic masses given in the periodic table of elements.

Divide the molar mass of the compound by the empirical formula mass. The result should be a whole number or very close to a whole number. Multiply all the subscripts in the empirical formula by the whole number found in step 2. A mole is a familiar unit of measure that measures the number of atoms and molecules in a bulk sample of matter. To put it another way, a mole is a unit of substance whose mass is equal to the mass in grams of pure 12C that contains exactly 12 atoms.

For this unit, "mole" means "large mass," which is consistent with the Latin connotation of the word. One of the most fundamental properties of matter, the number of atoms and molecules, is linked to a more easily observed macroscopic feature. Six moles is 60% of the inital moles in the container, so the final pressure will be 60% of the initial pressure.

The density of a certain gaseous fluoride of phosphorus is 3.93 g/L at STP. Calculate the molar mass of this fluoride and determine its molecular formula. Small particles suspended in a fluid medium exhibit random motion due to collisions with the surrounding molecules.

This effect, known as Brownian motion, occurs in all fluids and at all pressures and can be used as a measure of the number density of molecules in a gas. A pressure gauge using this principle can be considered a primary pressure standard since its calibration can be predicted only from the knowledge of the particle dimension and density. An outstanding feature is that it can determine pressures down to the lowest degree of rarefaction attainable under laboratory conditions. We can apply the Ideal Gas Law to solve several problems. Thus far, we have considered only gases of one substance, pure gases. We also understand what happens when several substances are mixed in one container.

From this equation, 1 mole of PH3 results in the formation of 1/z moles of gaseous Pz molecules and 3/2 moles of hydrogen gas, H2. Therefore from 2 moles of PH3 there would be formed 2 x 1/z moles of Pz and 2 x 3/2 moles of H2. You can adapt to the set of units you'd like to use just by changing the gas constant. Here are the constants and the units of pressure, temperature and volume that go with them. While, when using gas laws like Charles' law and the Gay-Lussac law, it's OK to use Celsius temperatures , it's important to use Kelvin temperatures in the ideal gas law.

When using the ideal gas equation, the unit of universal gas constant must be taken carefully. Because the value of R changes with the unit of pressure, temperature and volume. The molar volumes of all gases are the same when measured at the same temperature and pressure (22.4 L at STP), but the molar masses of different gases will almost always vary. Particles are in constant, random motion, and they collide with one another and the walls of the container in which they are enclosed.